1. Introduction

Concrete remains a foundational material in construction, playing a crucial role in the infrastructure development of many countries [1]. Since its introduction in the 1950s, prestressed concrete has become a cornerstone of the construction industry, offering numerous benefits such as reduced material use, lower transportation costs, and improved durability and service life of structures [2]. In particular, pretensioned prestressed concrete has emerged as an efficient structural material widely utilized in civil engineering projects. This method allows for quicker construction, high-quality fabrication, and optimal utilization of material properties while also addressing environmental considerations [3]. The bond between the strand and concrete is a critical component of this technology; without it, the intended prestress in the concrete members could not be realized, compromising the overall performance and durability of the structure. The bond behavior is typically characterized by the local bond stress-slip relationship and is influenced by several factors, including the properties of the reinforcing materials—such as bars, multiwire strands, or tendons—the characteristics of the concrete, the dimensions of the embedded reinforcement, environmental conditions, and the effects of cyclic loading [4].

The anchorage and development of prestressing force depend exclusively on the bond after the release of strands [5]. To characterize the bond behavior in pretensioning anchorage, two different bond situations should be considered due to the transverse deformations of the tendon. The "push-in" scenario occurs in the transfer length, where the tendon expands transversely upon prestress release. In contrast, the "pull-out", refers to the flexural length where the opposite occurs when the steel stress is increased due to loading [6]. The transfer bond forms at the release of the prestressing strand, transferring the prestress force from the strand to the concrete in the end zone. The transfer length is the distance needed for the strand to effectively transfer prestress to the concrete. The flexural bond activates with applied loads, increasing strand stress and causing concrete cracking. Its length extends from the end of the transfer length to the point where ultimate stress is achieved [7]. The bond resistance between the strand and surrounding concrete arises from adhesion, friction forces, and mechanical interaction due to the shape of the strand. In the transmission zone, this bond strength is further enhanced by the Hoyer effect, a phenomenon resulting from the tendon’s wedging mechanism [8]. The Hoyer effect increases bond capacity in the transfer length compared to the flexural bond length, resulting in a steeper gradient of pretensioning force [9]. Vázquez-Herrero et al. [10] conducted push-in and pull-out tests to examine bond strength in the transfer and flexural lengths, respectively. They found bond stress in the transfer length was 1.3–2.7 times higher than in the flexural length, attributed to the strand’s wedging effect observed in the push-in test (Hoyer effect) but not in the pull-out test.

The most accurate way to evaluate strand bond performance is through testing a full-scale beam, but this method is often not cost-effective or time-efficient. As an alternative, pull-out tests have been developed and are commonly used to approximate real bond behavior [11]. Among these, two primary types are recognized: the simple (untensioned) pull-out test and the tensioned pull-out test [12]. Regarding transfer bond, the simple pull-out test, while conservative, does not account for the frictional bonding benefits seen in tensioned scenarios due to the Hoyer effect, as it does not incorporate the lateral dilation of the prestressing tendon caused by the Poisson effect [10]. To address this, more complex tensioned pull-out tests, such as those proposed by Cousins et al. [13] and Abrishami and Mitchell [14], have been developed. These tests involve pretensioning the strand before casting the concrete and measuring bond forces through various methods, such as push-off loads or tension release via threaded screws. Jiang, X. et al. [11] conducted a comparative study on transfer bond using both untensioned and tensioned pull-out tests with different embedment lengths and found that tensioned tests generally produced higher pull-out forces, especially with shorter embedment lengths. This suggests that simple pull-out tests might underestimate bond strength, particularly for pretensioned strands where frictional effects are significant. Regarding flexural bond, the tensioned pull-out test developed by Abrishami and Mitchell [14] also allows for the complete bond stress-versus-slip response to be determined. This test involves pretensioning the strand before casting the concrete and measuring bond forces through increased tension via threaded screws. However, the tensioned pull-out test can be challenging to execute and sometimes yields inconsistent results, likely due to the complexities involved in the testing process [15].

There has been significant advancement in numerical modeling within this area [16,17]. This approach is frequently utilized in design processes due to the challenges, costs, and time associated with experimental methods. Moreover, predictions from numerical models tend to correlate well with experimental results [18]. However, finite element (FE) modeling of the bond interface between concrete and reinforcement is still relatively underrepresented in the literature. Current numerical models often do not adequately incorporate bond effects, and analytical methods generally address bond interactions in a simplified manner. Two primary approaches exist for modeling the pull-out behavior of a bar or anchor from concrete: one uses a one-dimensional bar with bond characteristics, while the other employs volume and three-dimensional (3D) interface elements [19]. The most commonly used software packages for the nonlinear analysis of reinforced concrete structures include ATENA [19], ANSYS [20], and ABAQUS [21]. Notably, ATENA [19], with its sophisticated constitutive models, provides valuable support for experimental investigations and the development of innovative solutions in the study of reinforced concrete members [22].

Nardin et al. [23] examined the steel-concrete interface using ANSYS and ABAQUS. They focused on 10 mm deformed bars embedded in concrete with a compressive strength of 30 MPa. The study utilized non-linear material models for both concrete and reinforcing bars, including a representation of their interaction. The findings revealed that ABAQUS was more effective in predicting the ultimate load, while ANSYS provided a better depiction of the behavior leading up to the peak load. In a similar study, Abed et al. [24, 25] simulated pull-out tests using the ATENA 3D software, employing a one-dimensional bar model. They defined a bond stress-slip curve in the software based on pull-out test experiments tailored to the specific concrete and reinforcing bar materials. The numerical results were closely aligned with the experimental data, accurately reflecting the observed behavior. Carroll et al. [26] used numerical modeling to accurately predict the behavior of structural members, validating their results against experimental tests. Their method involved using a matrix of solid elements to represent the concrete, truss elements for the prestressing strands, and nonlinear springs at the interface between the strands and the concrete. This approach proved effective and accurate in simulating prestress transfer, including bond-slip behavior, and closely matched experimental data in terms of predicting end slip, strand force development, transfer length, and concrete surface strains. Zhan et al. [27] investigated the bond properties of 17.8 mm diameter steel strands embedded in Ultra-High Performance Concrete using pull-out tests. They created FE models to simulate the pull-out behavior, which showed good agreement with the experimental load-slip curves, with discrepancies in peak load predictions within 7%, indicating high model accuracy. Tavares et al. [28] explored the bond behavior for different bar diameters using simulated pull-out tests with ATENA 2D, finding their numerical results to be consistent with experimental outcomes. Additionally, Seok et al. [29] developed a FE model to simulate the bond behavior in high-strength concrete beams. Their model successfully captured the pattern of concrete splitting-crack development, as confirmed by comparison with actual test results. Tsiotsias et al. [30] conducted simulations of various pull-out test setups using 3D modeling components for both the bar and concrete. They employed isoparametric gap elements coupled with a pressure-sensitive Mohr–Coulomb interface to model the interaction, focusing on 16 mm reinforcing bars in 25 MPa concrete. Burdziński et al. [31] examined the impact of bar diameter on bond behavior through a combination of experimental, analytical, and numerical methods. They used the concrete damaged plasticity material model and contact cohesive behavior approach to represent the concrete-bar interface. Their research, which involved C35/45 grade concrete and B500SP bars, found a good agreement among FE models, analytical methods, and experimental data, confirming consistent bond behavior across different bar diameters.

The current debate in the field revolves around the accuracy of simple versus tensioned pull-out tests in representing the bond behavior of pretensioned members. While some researchers have employed simple pull-out tests, others have developed tensioned pull-out tests to more accurately represent the bond behavior in pretensioned members. Those who favor tensioned pull-out tests argue that simple pull-out tests do not accurately capture the bond behavior in pretensioned members. Conversely, proponents of the simple pull-out test believe it provides sufficient insights and point out that the tensioned pull-out test can be challenging to execute and sometimes yields inconsistent results, likely due to the complexities involved in the testing process. To study the bond behavior between strands and concrete in the flexural zone, it is crucial to identify the most suitable testing method, with both pull-out tests and tensioned pull-out tests being commonly used. As discussed, several experiments have been successfully simulated using various software, where the numerical results closely align with those of experimental tests. To address these contrasting views, this research will conduct a numerical study to examine the differences in bond strength between simple and tensioned pull-out tests, with a specific focus on the flexural bond. Additionally, the study will investigate the impact of varying tension ratios on bond strength.

2. Methodology

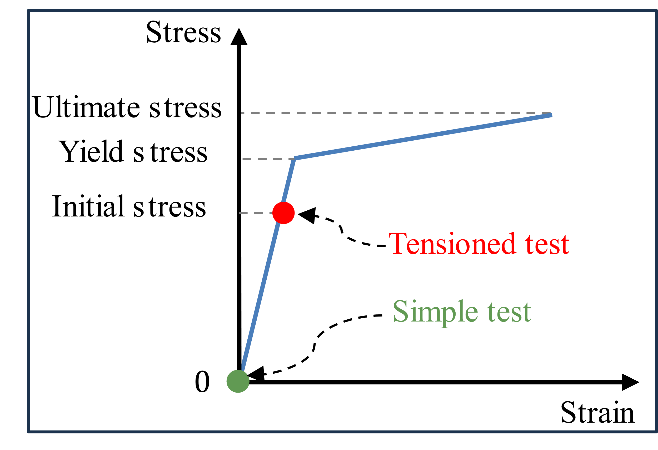
In pretensioned members, the flexural bond mechanism is activated under applied loads, causing the stress in the strand to increase. This "pull-out" scenario involves a reduction in the strand's diameter due to Poisson's effect as the steel stress rises with loading. At the onset of loading, the strand already possesses an initial stress due to prestressing. In the tensioned pull-out test, as the load increases, the stress in the strand rises further until bond failure occurs, without reaching the yield strength [32]. In simple pull-out tests, the strand is initially unstressed, which does not accurately represent the behavior observed in pretensioned members, where an initial prestress is present [33] (Figure 1). To more accurately simulate the conditions in pretensioned members, researchers have developed tensioned pull-out tests. In these tests, the strand is first tensioned to an initial stress level prior to the casting of concrete, thereby reflecting the initial conditions found in actual structures. The testing then commences with the strand already under this initial stress.

In this study, numerical modeling is employed to enhance the understanding of bond behavior in pretensioned members, specifically focusing on the flexural bond. The FE model used for this purpose was developed to simulate pull-out tests, incorporating 3D elements for both the concrete and the reinforcing bar. The bonded interface was modeled using isoparametric gap elements, which were equipped with a pressure-sensitive Mohr–Coulomb frictional interface. Part of this model, including the simple pull-out test, has been validated in previous research by the authors [17], which studied bond behavior in seven-wire strands and lightweight aggregate concrete. For a more detailed validation, reference can be made to that article. This study extends the analysis by focusing on tensioned pull-out tests, introducing a parametric study of varying tension ratios (25%, 50%, 85%, 95%, 100%, and 103% of the yield strength of the strand) to assess their impact on bond strength. The comparison between simple and tensioned pull-out tests highlights the influence of pre-applied tension on bond performance.

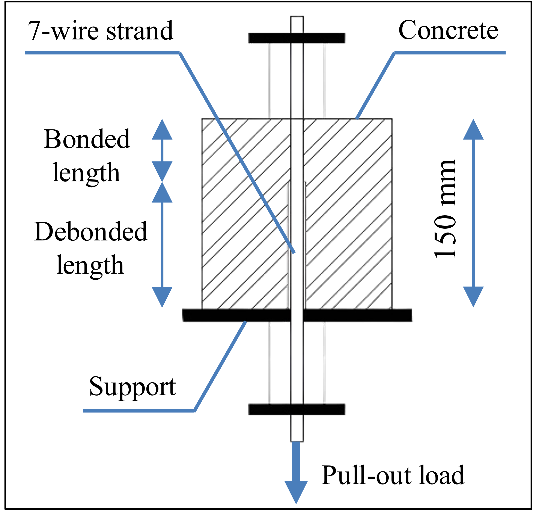
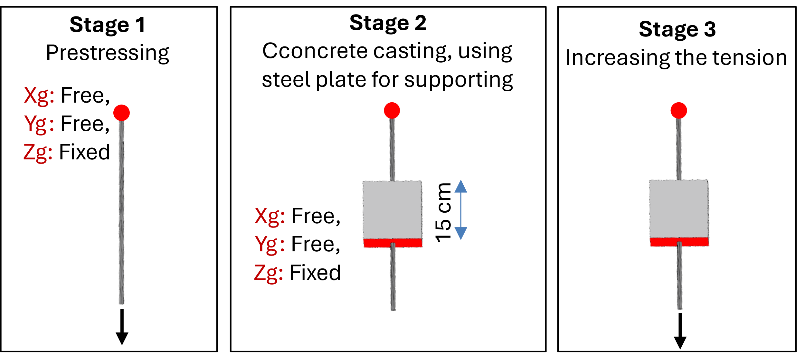
The pull-out test was simulated using ATENA 3D software, adhering to the RILEM recommendations for steel reinforcement [33, 34]. The test setup involved preparing a cubic specimen measuring 15 cm, with the reinforcement embedded within the cube. The embedment length was set at five times the diameter of the reinforcement, as illustrated in Figure 2. The rationale for selecting a short embedment length lies in the non-uniform distribution of bond stresses along the length, which tends to increase with the bonded length. Therefore, a shorter embedment length was chosen to achieve a more consistent bond stress-slip relationship [35]. ATENA 3D was particularly suitable for this analysis due to its specialized design for concrete, offering user-friendly features and appropriate default values [19]. The tensioned pull-out test was conducted in three distinct stages, as depicted in Figure 3. In the first stage, the strand was pretensioned to specific ratios of its yield strength. Following this, the concrete was cast around the pretensioned strand while maintaining the applied tension. In the final stage, the tensioning force was gradually increased until bond failure occurred.

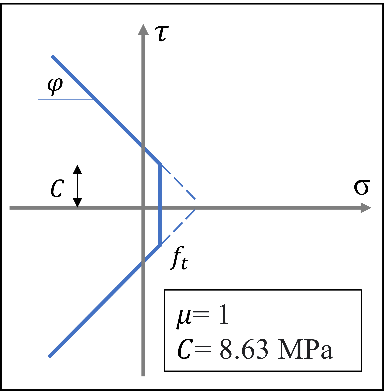
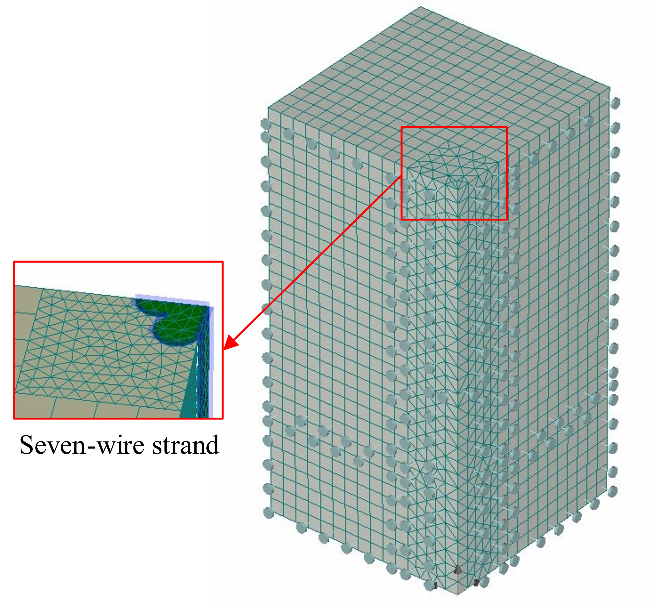
The specimen detailed in a previous reference [4] utilized lightweight concrete with an average compressive strength of 41.65 MPa and an average tensile strength of 2.58 MPa. The concrete had an elastic modulus of 20.211 GPa and a density of 1744 kg/m³. The reinforcement used was a seven-wire strand with a 12.7 mm diameter and a grade of 1860 MPa. This strand was an uncoated low-relaxation strand, featuring a nominal cross-sectional area of 97.65 mm² and an elastic modulus of 196.5 GPa. The properties of both the concrete and the reinforcement were input into the software for accurate simulation. The interface elements (CCIsoGap) were chosen to simulate the contact surfaces between the concrete and the strand, with their properties illustrated in Figure 4, where μ represents the friction coefficient, and C denotes cohesion [17]. The reinforcement was modeled using solid elements with the material model CC3DBiLinearSteelVonMises, employing the Von Mises Plasticity Model. This approach allows for the simulation of the reinforcement as an elastic-plastic material with linear hardening properties. For the concrete, the material model CC3DNonLinCementitious2, as specified in the ATENA Manual [19], was used. The concrete was represented with 3D solid brick elements (CCIsoBrick) with reduced integration (8 nodes), while 3D solid tetrahedral elements (CCIsoTetra) with reduced integration (4 nodes) were applied to the concrete adjacent to the strand (refer to Figure 5). The concrete zone was modeled to be approximately double the size of the bar. To reduce computational effort, only one-quarter of the specimen section was modeled, utilizing symmetry and enforcing boundary lateral restraints on the symmetry planes. Vertical support was also provided at the bottom surface of the concrete cube. The mesh size used was 3 mm, ensuring accuracy in the simulation. The analysis employed the Standard Newton-Raphson iteration method with displacement load, using an increment of 0.01 mm until specimen failure, with the load applied at the bottom surface of the reinforcement.

3. Results

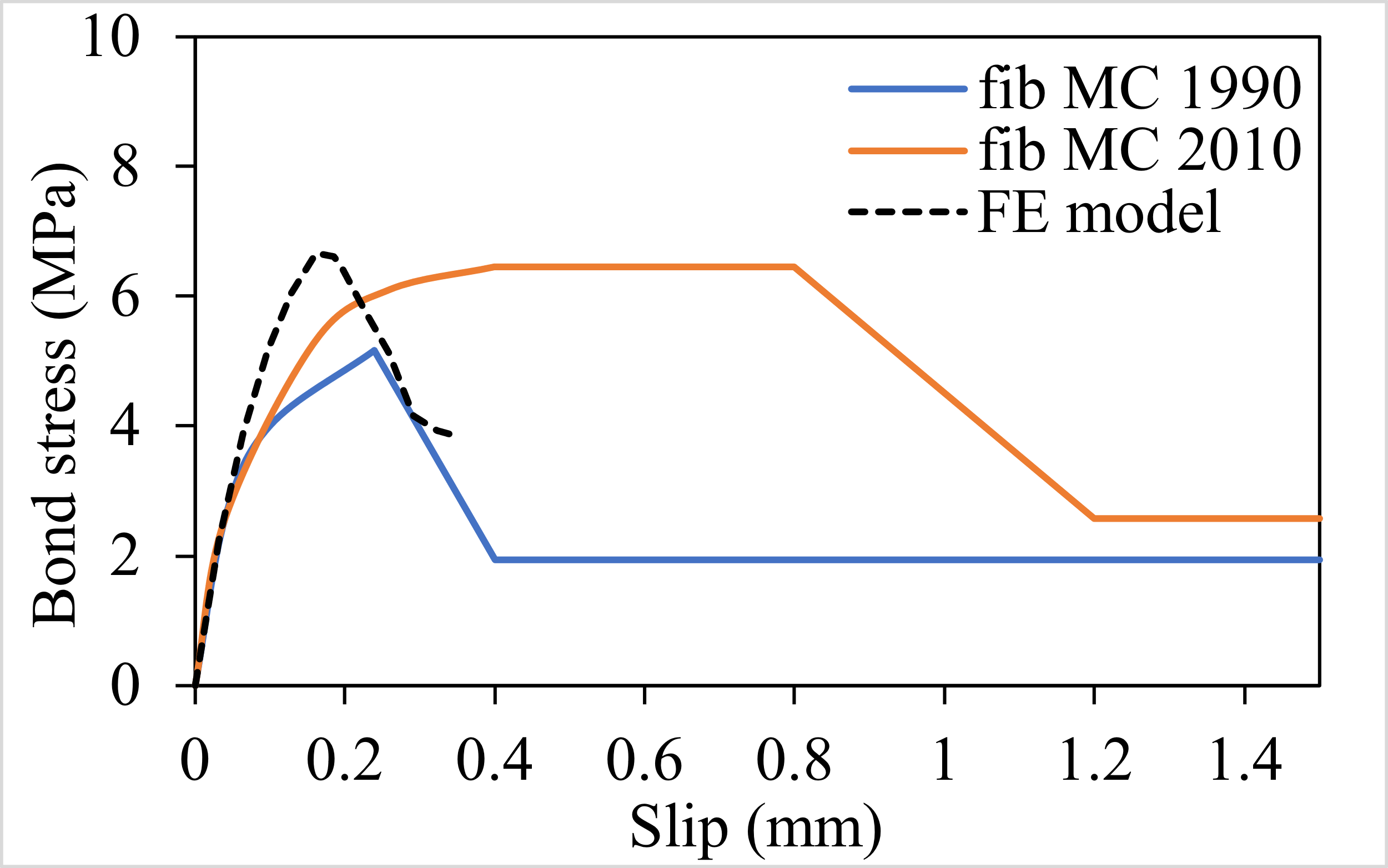
The simple pull-out test was initially validated against previous research, demonstrating consistent bond behavior under untensioned conditions. For a more detailed validation, refer to [17]. Figure 6 presents the bond stress–slip curve, comparing the FE model with the fib MC1990 [36] and fib MC2010 [6] models. The close alignment between these curves confirms the validity of the FE model for the simple pull-out test.

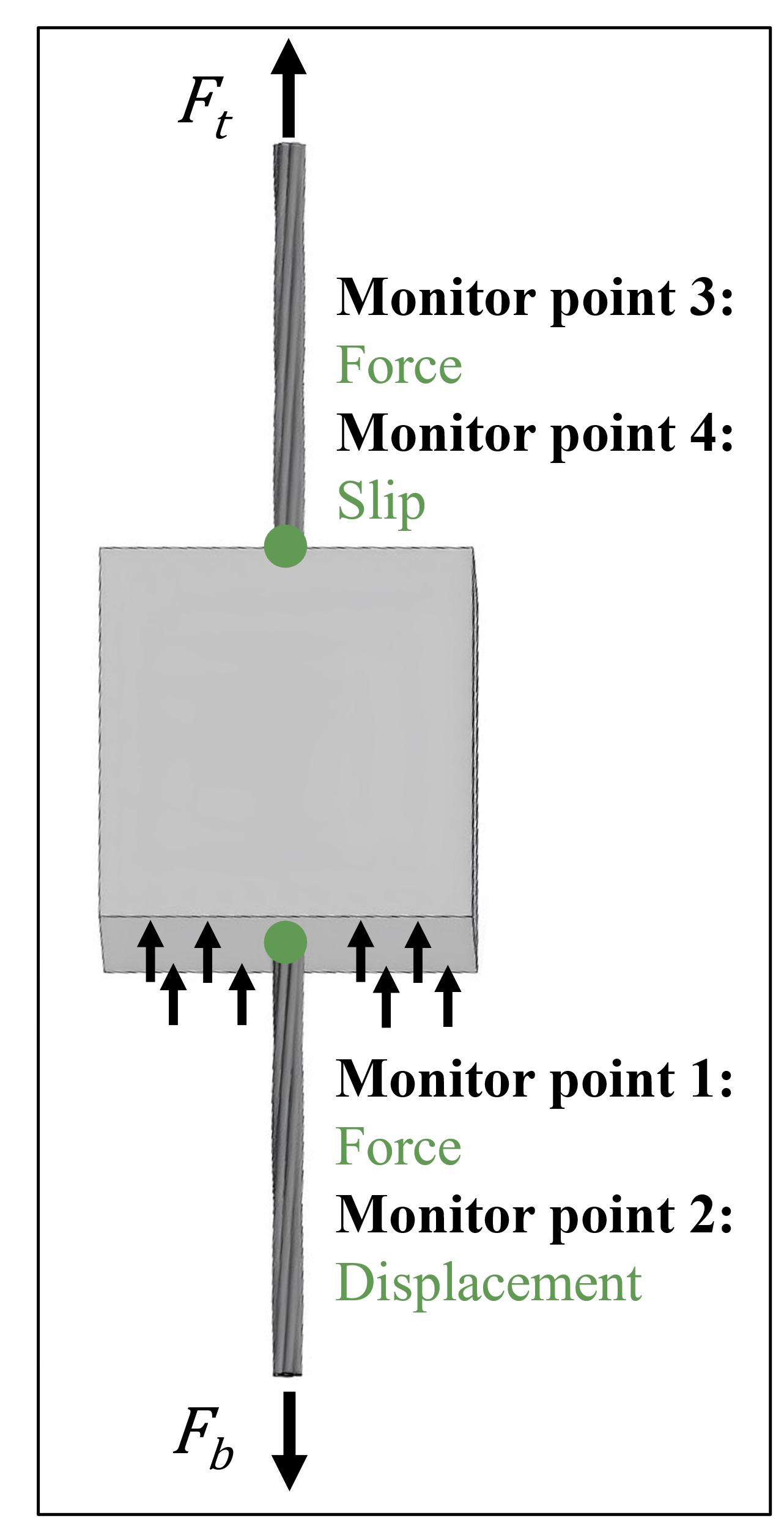
The monitored data from the numerical model provide crucial insights into its performance. Four monitoring points were strategically chosen: the first and second points tracked the force applied and its displacement of the strand at the concrete cube bottom, while the third and fourth points measured the force and the slip of the strand at the concrete cube top, as illustrated in Figure 7. This setup enabled the calculation of stress and the derivation of the stress-displacement curve for the strand, which in turn allowed for the determination of bond stress at various slips.

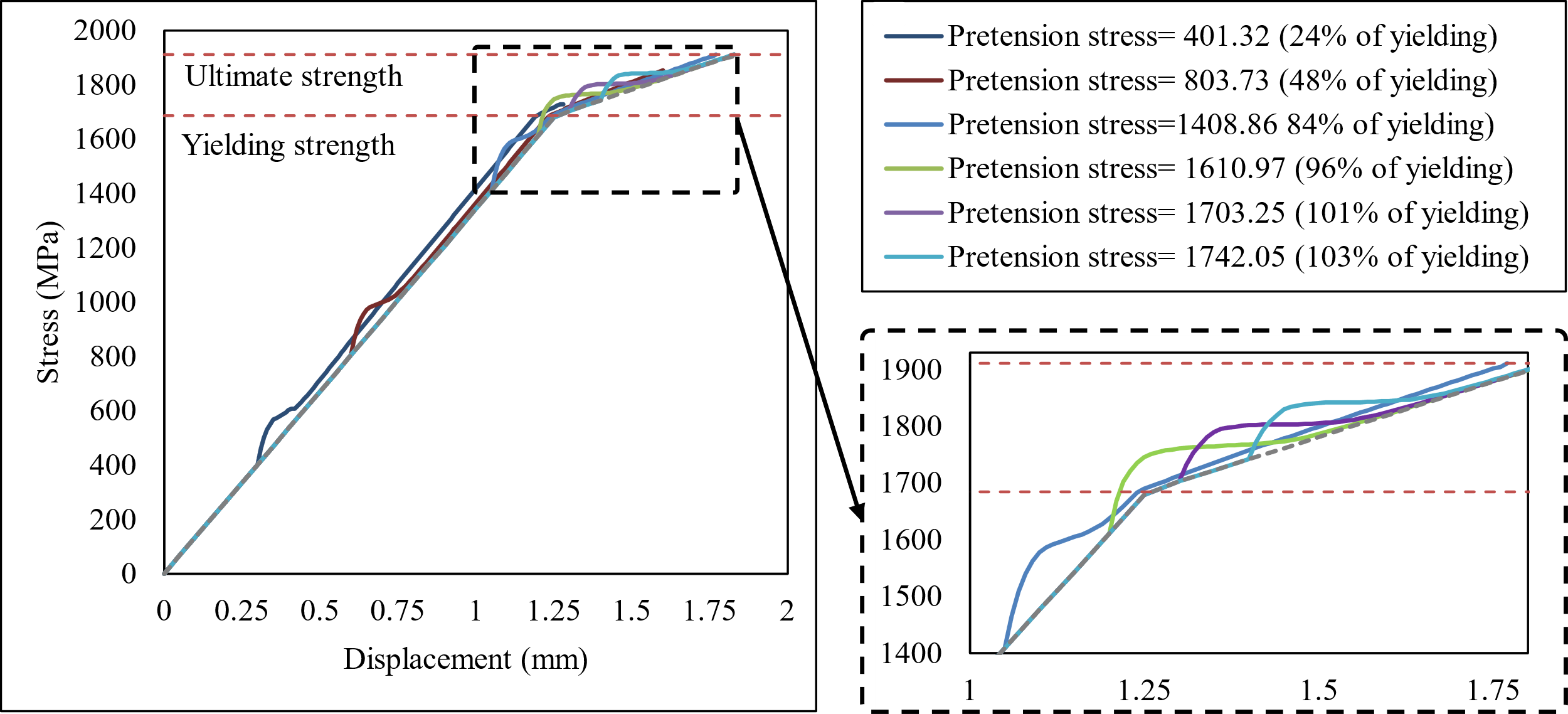
For the tensioned pull-out tests, six FE models were simulated, corresponding to varying tension ratios of 25%, 50%, 85%, 95%, 100%, and 103% of the yield strength of the strand. Figure 8 presents the results from these simulations. Each case showed a stress-displacement curve that initially followed the pure tension relationship for the strand. Upon the application of force with the concrete cube in place, the stiffness increased until bond failure occurred, after which the curve returned to follow the stress-displacement pattern of the strand. The force continued to increase until the strand ultimately ruptured.

To further analyze the results, bond stresses were computed for each case, and the bond stress-slip curves were plotted in Figure 9, which includes data from both the simple pull-out test and the tensioned pull-out test specimens. The results, including tensioning stress, displacement, and bond strength, are detailed in Table 1.

For the simple pull-out test, the bond strength was found to be 6.867 MPa, with a relative slip of 0.147 mm. For the tensioned pull-out test, the slip values associated with bond strength were fairly consistent, averaging around 0.159 mm across all FE models. Bond strength for the tensioned pull-out tests was calculated using Eq. 1, which divides the difference in force applied at the bottom (F_b) and top (_t) of the strand by the lateral contact area of the embedment length (l_b), where ∅ represents the nominal diameter of the strand.

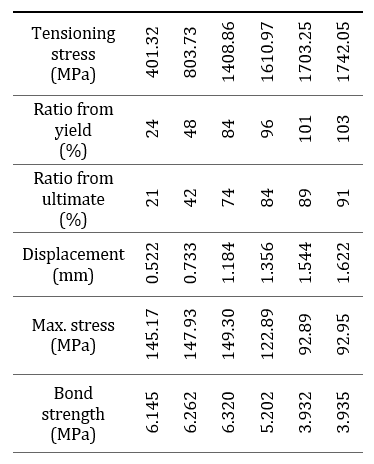
It was found that the simple pull-out test resulted in slightly higher bond strength compared to the tensioned pull-out test for models conducted below the yield strength threshold. Additionally, when comparing to the tensioned pull-out test for models below the yield strength, a significant reduction in bond strength was observed in two cases: first, when the pull-out test was initiated just prior to the onset of yielding and continued as yielding occurred, resulting in an 17% decrease in bond strength; and second, when the pull-out test began after the strand had yielded, leading to a notable 37% decrease.

(1)

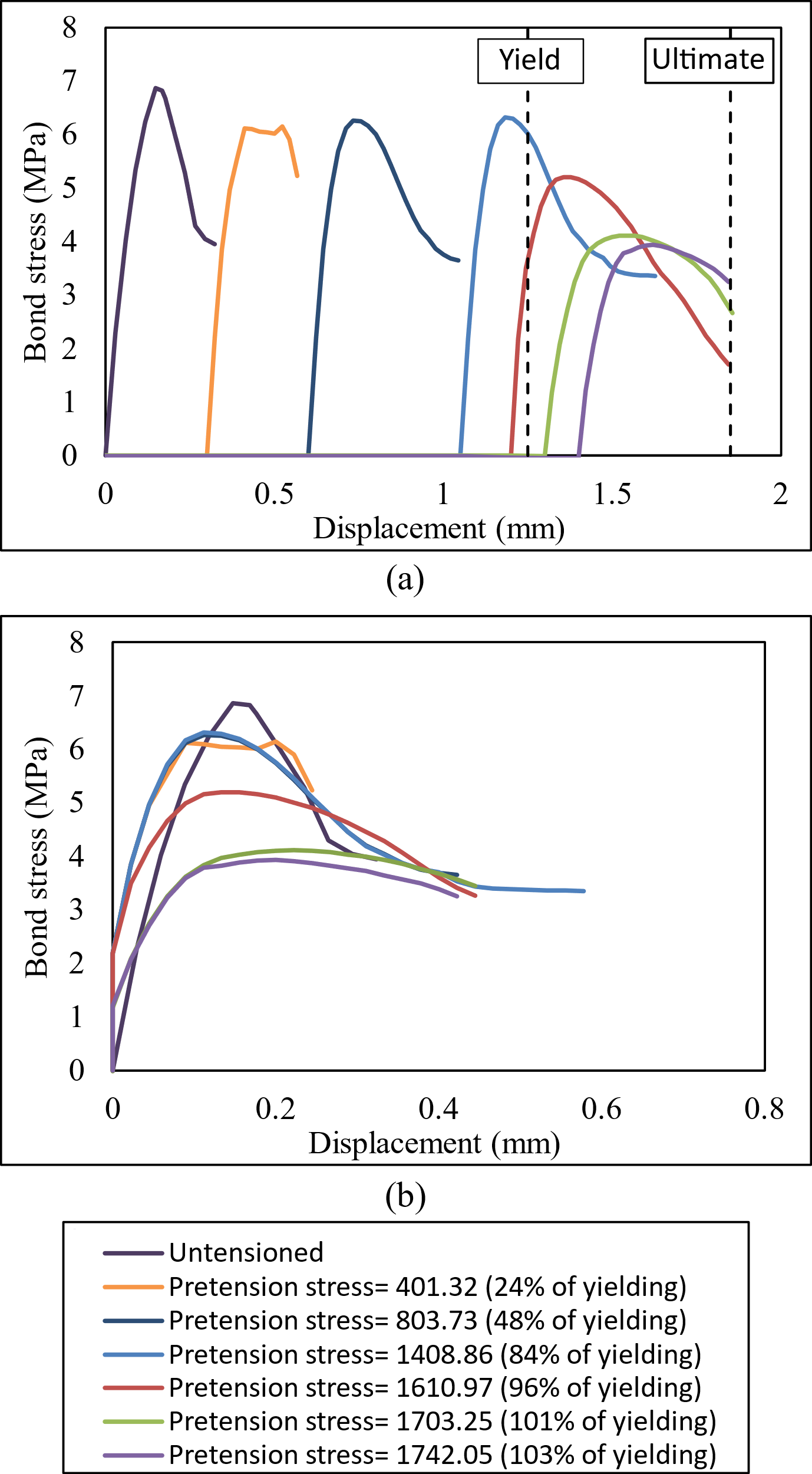
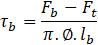
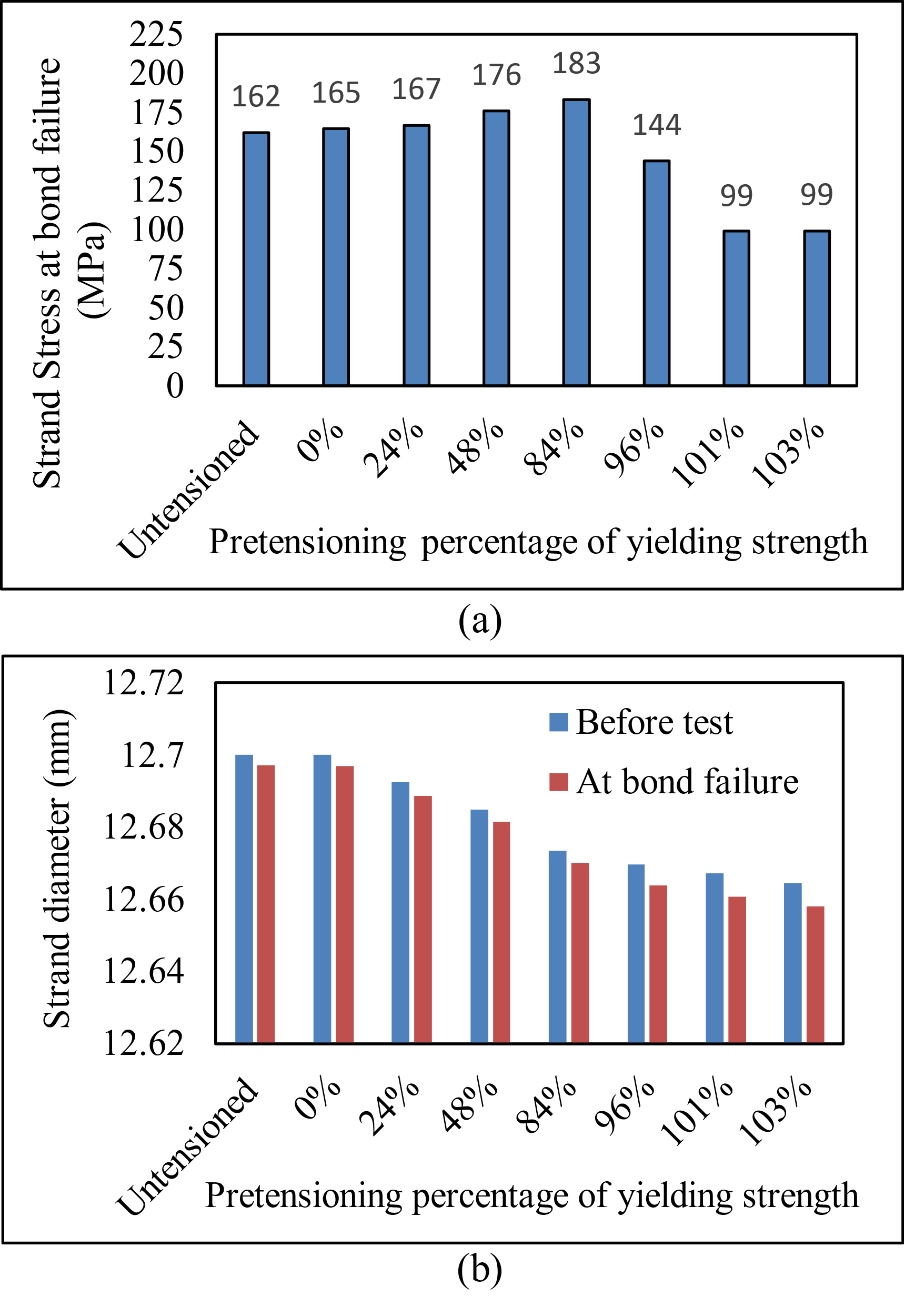
For a more comprehensive understanding of the variations in bond strength across the different models, it is essential to examine the strand stress at failure and assess changes in the nominal diameter of the strand at failure. This assessment includes comparing the strand's nominal diameter before and after the test to evaluate the effect of Poisson's ratio, as shown in Figure 10. The Poisson effect is one factor influencing the results, alongside the yielding of the strand, particularly in models tested at or beyond the yield strength. The results indicated that increasing tensile stress led to a reduction in the strand diameter due to the Poisson effect, which diminished the contact area and friction between the strand and concrete, consequently lowering bond strength. The reduction in strand diameter observed between the pre-test measurement and at bond failure was approximately 15% greater in tensioned tests conducted below the yield strength threshold compared to untensioned tests. This difference is attributed to the higher stress levels in the strand at failure. However, when the test was initiated just prior to or after the onset of yielding, this reduction was substantially larger, around 55% greater than in untensioned tests.

In conclusion, the simple pull-out test can effectively investigate the bond strength of pretensioned members in the flexural zone as found by Gessner et al. [12]. The results of the simple pull-out test in this study closely aligned with those of the tensioned pull-out test when the pretensioning force remained below the yield strength threshold, a typical condition in pretensioned members. Furthermore, the study highlighted the impact of varying tension ratios on bond strength, demonstrating a clear correlation between tension levels and bond performance.

4. Conclusion

This study conducted a detailed numerical investigation to enhance the understanding of bond behavior in pretensioned members, focusing specifically on the flexural bond. The FE model, validated in previous research, was utilized to simulate both simple and tensioned pull-out tests, incorporating seven-wire strands and lightweight aggregate concrete. Besides, the research investigated the effects of varying tension ratios on bond strength, with tensioned pull-out tests conducted at 25%, 50%, 85%, 95%, 100%, and 103% of the yield strength of the strand. Models for the concrete and reinforcement bars utilized 3D elements, with the interface between the two materials simulated through 3D isoparametric gap elements featuring a pressure-sensitive Mohr-Coulomb frictional interface. The findings revealed several key insights:

• The untensioned test yielded a higher value, approximately 8% greater than the tensioned test, when conducted below the yield strength threshold.
• The pretensioning percentage exhibited no discernible impact when it remained below the yield strength threshold.
• The bond strength showed an 17% decrease when the pull-out test was initiated just prior to the onset of yielding and continued as yielding occurred during the test.
• A notable 37% decrease in bond strength was observed when the pull-out test commenced after the strand had yielded.
• Across all models of the tensioned pull-out test, the slip values were relatively consistent, with an average of approximately 0.159 mm. This consistency indicates that despite variations in initial prestress, the relative slip behavior remained stable.
• The decrease in strand diameter, observed between the pre-test measurement and at bond failure, was slightly greater in tensioned tests conducted below the yield strength threshold, approximately 15% more than in untensioned tests. This disparity is attributed to the higher stress in the strand at the point of bond failure.
• However, when the test was initiated just prior to or after yielding, this reduction was substantially larger, around 55% greater than in untensioned tests.

References